Chapter 7 Part 2 Conservation of Energy Including Examples with Friction

Prof. Clements Notes/Hints for Physics Courses

Chapter 7 Part 2 Open Stax College Physics or Most Introductory Physics Courses

Friction, Conservation of Energy

Terms you should know at the end of this unit: nonconservative, conservation of energy, efficiency

Friction - Nonconservative Forces

For a nonconservative force the path of motion makes a difference in the work done and the change in energy of the system. The direction of the force of friction is always opposite to the direction of velocity, when the object is in motion. The direction of the force of friction is always opposite to the direction of the net applied force, when the object is at rest. The full friction force, not just some component in the X direction for example, is used to calculate the work done by friction. The work done by friction is equal to the force of friction multiplied by the distance traveled, not by the straight line displacement.

Conservation of Energy

For the mathematical statement of Conservation of Energy I will use:

KE₁ + PE₁ + Work_friction = KE₂ + PE₂ .

KE is the energy for objects in motion, ½ m V² .

PE is the energy by virtue of position or configuration:

mgh for vertical problems, ½ k X² for springs.

Work_friction will be a negative number as friction takes away energy.

I would recommend that you watch this video: Mechanical Universe #13 Conservation of Energy (28 minutes).

https://www.youtube.com/watch?v=8BDHN2bY1bg

Work Done by Nonconservative Forces

The concept here is that the amount of energy available at the start of the problem is not all available for KE and PE at the end of the problem because work is done by dissipative forces. KE₁ + PE₁ is the supply of energy at the start of the problem. KE₂ + PE₂ is the amount of energy in the system at the end of the problem.

KE₁ + PE₁ + Work_friction = KE₂ + PE₂ The left side calculates the supply of energy at the start as adjusted by the work done by friction. The work done by friction is a negative number. The right side calculates the supply of energy at the end. If friction is present in a problem then KE₂ + PE₂ < KE₁ + PE₁ .

Note that the PE terms are actually the work done by the conservative forces. It is just more convenient to express these energies as PE rather than Work calculations.

Why is the work done by friction always a negative number?

Answer: The friction force arrow is a direction 180 degrees opposite to the direction of the velocity. Work = F cos( θ) d . The value of cos(180 degrees) is -1.

e.g. #1 A 10 kg block of wood is resting on an inclined plane that has an angle of 62 degrees to the horizontal. The coefficient of friction is 0.0. The wood is 1.4 meters up from the bottom of the plane as measured along the direction of the plane. The wood is released from rest. Calculate the speed of the wood just before it reaches the bottom of the plane.

e.g. #2 This problem is a repeat of e.g. #1 but now with friction on the plane.
   A 10 kg block of wood is resting on an inclined plane that has an angle of 62 degrees to the horizontal. The coefficient of static friction is 0.22. The coefficient of kinetic friction is 0.18. The wood is 1.4 meters up from the bottom of the plane. The wood is released from rest. Calculate the speed of the wood just before it reaches the bottom of the plane.

e.g. #3 An object (mass m) slides down a ramp (ramp angle theta) and compresses a spring (force constant k) on the ramp.  Find the general expression for the distance the spring is compressed.  Ignore friction.

e.g. #4 An object (mass m) slides down a ramp and then moves around a circular loop (radius r).  Determine the expression that allows you to calculate the starting height (above the exit of the loop) for the object.  Ignore friction.

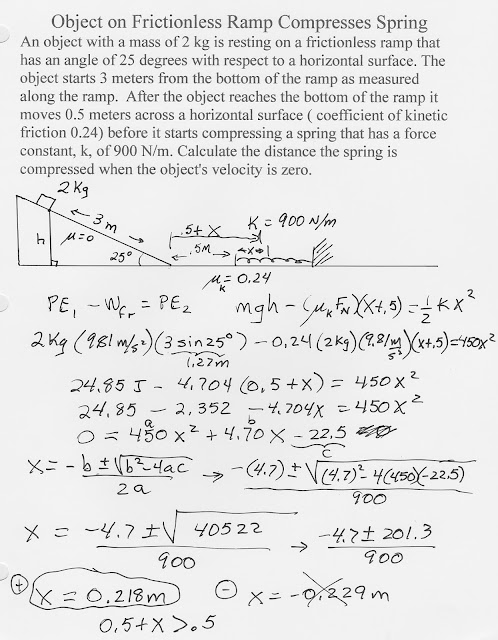
e.g. #5 An object (2 kg) starts a distance of 3 meters from the bottom of a ramp (25 degrees) as measured along the ramp. The object slides down a ramp and then moves on a horizontal surface that has friction (coefficient of friction 0.24). On this horizontal surface the object compresses a spring (force constant 900 N/m).  Calculate the distance the spring is compressed.

Watch YouTube videos and ask your instructor if you have questions on this material.

Efficiency

Efficiency = Work Done by System/ Energy Put Into System

e.g. A new car engine might have an efficiency of 30% (according to the table in the OpenStax Physics textbook). Suppose you put $40 of gas into the tank of the car. This is the value of the energy put into the car. What is the dollar value of the work done by the engine?

Answer: 0.30 = W / $40 so W = 0.30 * $40 or $12 ! The other $28 basically went out the exhaust pipe.

For a total car system there is energy loss in the brakes, transmission, wheels, etc., so the overall efficiency drops to 20% or less.

Why do hybrid cars (electric motors on wheels, large battery, small engine, etc.) have higher efficiencies than other cars?

Answer: A large improvement in efficiency comes in the braking system. In a hybrid car, when you step on the brake pedal the motors that drive the wheels become electrical generators. During the braking process the batteries are recharged. On regular cars the braking process creates thermal energy that escapes into the environment and can’t be used in the future to accelerate the car.

Copyright© 2017 by Greg Clements Permission is granted to reproduce this document as long as 1) this copyright notice is included, 2) no charge of any kind is made, and, 3) the use is for an educational purpose. Editing of the document to suit your own class style and purposes is allowed.